de Broglie - Matter Waves & Origin of Matter
In 1929 Maurice de Broglie established the hypothesis that the dual wave-particle behaviour of radiation applies equally well to matter33. Just as a photon has a light wave associated with it that governs its motion, so a material particle (e.g. an electron) has an associated matter wave that governs its motion. He proposed that the wave aspects of matter are related to its particle aspects in exactly the same quantitative way that is the case for radiation. According to de Broglie, for matter and for radiation alike, the total energy E of an entity of mass m is related to the frequency ɣ of the wave associated with its motion by the equation E = m c2 = h ɣ where h is Plank's constantand c is the speed of light, and further the wavelength λ is related to the momentum p = mv by the equation λ = h/p where v is the velocity of the entity.
Physics in 5 Dimensions introduces the hypothesis that all stable matter (particles and larger bodies) move along closed paths with a common constant velocity. In general the shape of the path (orbit) will be an ellipse or as a special case a circle; small for an electron and large for a planet. Therefore all particles and bodies have an intrinsic value of angular momentum arising from a closed path of nominal radius R4 with orbital velocity34 v4. In a 5-dimensional local space the angular momentum is given by the expression h5 = 2𝝅 m v4 R4 where m is the non-varying mass of the body (equivalent to the “rest” mass mo of classical physics) and we note that λ = 2𝝅 R4. This expression applies to all particles and bodies in 5-dimensional space and we get he = 2𝝅 me v4e R4e for the special case of an electron orbiting the nucleus of a hydrogen atom where he is Planck’s constant and me, v4e and R4e are respectively the mass, orbital velocity and radius of orbit of the electron.
A further hypothesis of Physics in 5 Dimensions states: The angular momentum h5 and mass m of a particle or body is constant and uniquely defines the energy and permitted motion of the body in 5-dimensional space. This implies that the product v4 R4 is also constant so that for example if the orbital velocity is the speed of light c with an orbit of radius Rc then R4 v4 = Rc c.
The form of the expression h5 = 2𝝅 m v4 R4 allows us to consider including the path of the matter wave in our equations of motion by defining R5 to be the radius of a closed path followed by the matter wave with velocity w4. We will also assume that the angular momentum is conserved for the matter wave, which is the case if we define R4 v4 = R5 w4. This is a fundamental expression of Physics in 5 Dimensions.
From de Broglie’s postulate we also have the relationship v4 w4 = c2 and from the above expressions it follows that the matter wave velocity w4 = ɣ λ4. We note the hypothesis of Physics in 5 Dimensions that the frequency ɣ in the frame of reference of the object is constant.
Superposition of seven cosine waves35
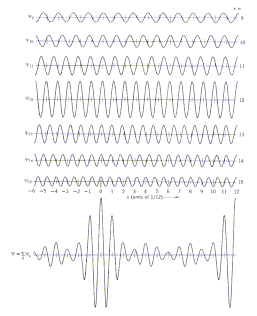
de Broglie showed that the superimposition of many sinusoidal matter waves produces a group of waves (lower plot in figure) to which we can assign the de Broglie - Einstein relations of energy E, momentum p = m v4, wavelength λ4 and frequency ɣ.
By using a solution to de Broglie’s postulate where the prime velocity vectors for the propagation of matter waves and the groups of waves is the velocity of light c, the two vectors then match all the criteria of the de Broglie theory and 5-dimensional space and the matter waves can be considered as the source of a group of waves of energy E with an implied mass m = E /c2.
As we now have matter waves with the velocity of light, a further hypothesis of Physics in 5 Dimensions follows: The origin of all matter is electromagnetic radiation in the form of many waves of different wavelength producing groups of waves with particle-like-nature (although the mechanism for doing this has not yet been considered).
Using the de Broglie expressions in the manner described also leads to expressions of Physics in 5 Dimensions similar to those of attractive forces in classical physics36 and we find that R5 c2 = R4 v42. The matter waves of all objects in a 5-dimensional local space orbit a common radius R5 with radial velocity w4 at the centre of the local space, while at the same time the associated objects (groups of waves) are orbiting on a centred common axis each with their own radius R4 and orbital velocity v4.
From classical physics we have R4 v42 = P M4 where P is a constant of dimensions and M4 is a body of mass M4 assumed to have an attractive force causing objects to rotate around it. The presence of the common expression R5 c2 = R4v42 in both the equations of 5-dimensional space and for an orbiting object involving an attractive force of 4-dimensional space leads us to consider the following hypothesis: The shape of the local space of an object of mass m is dependent on its angular momentum, the radius R5 linked with a central space around which the matter waves of all objects in the local space orbit and coincident with a core body of mass M4. This raises the intriguing idea of the matter waves of all the objects creating the body of mass M4 or vice versa, and the chicken & egg question of which came first?
While R5 and M4 are mathematically related using a constant of dimensions P we have not established any other Physics in 5 dimensions link between M4 and P. The 5-dimensional equations of motion have the same form as the classical physics equations involving forces of attraction, however the origin of matter and the matter waves in the 5-dimensional model replace the need for the attractive force of gravity in the case of large mass objects, such as the earth moving around the sun, and the electrostatic Coulomb force in the case of atomic particles, such as electrons moving around a nucleus.
The behaviour of both Particles in Quantum Physics and the Larger Bodies of the Universe are determined by 5-dimensional space theory. In all cases the expressions used for motion and energy are identical.
References:
(33) de Broglie’s “ The wave nature of the electron” – Nobel Lecture December 12, 1929 https://www.nobelprize.org/uploads/2018/06/broglie-lecture.pdf
(34) The magnitude of v4 is defined to be the scalar velocity v of an object as viewed by an observer in classical physics
(35) Ref. Fig 3.9 Quantum Physics, Robert Eisberg & Robert Resnick, John Wiley & Sons Inc., ISBN 0-471-87373-X
(36) electrostatic attraction in the case of electrons in atoms and gravity in the case of large bodies such as the planets and their sun
Select another Famous Physicist and Physics Topic of interest to you
The Book by Alan Clark- Physics in 5 Dimensions - is also available as a PDF file to members of ResearchGate here.
